DSPRelated.com
Free Books
   Free Books    Physical Audio Signal Processing  

Vector Wavenumber

Mathematically, a sinusoidal plane wave, as in Fig.B.9 or Fig.B.10, can be written as

$\displaystyle p(t,\underline{x}) \eqsp p_0 + A\cos\left(\omega t - \underline{k}^T\underline{x}+ \phi\right), \quad \underline{x}\in{\bf R}^3 \protect$ (B.48)

where p(t,x) is the pressure at time $ t$ (seconds) and position $ \underline{x}\in{\bf R}^3$ (3D Euclidean space). The amplitude $ A$, phase $ \phi$, and radian frequency $ \omega $ are ordinary sinusoid parameters [451], and $ \underline{k}$ is the vector wavenumber:

$\displaystyle \underline{k}\eqsp \left[\begin{array}{c} k_x \\ [2pt] k_y \\ [2p... ... \cos{\beta} \\ [2pt] \cos{\gamma}\end{array}\right] \isdefs k\,\underline{u}, $

where
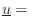  • $ \underline{u}= $ (unit) vector of direction cosines
  • $ k = 2\pi/\lambda = $ (scalar) wavenumber along travel direction
Thus, the vector wavenumber $ \underline{k}= k\,\underline{u}$ contains
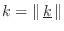
  • wavenumber along the travel direction in its magnitude $ k=\left\Vert\,\underline{k}\,\right\Vert$
  • travel direction in its orientation $ \underline{u}= \underline{k}/k$
Note that wavenumber units are radians per meter (spatial radian frequency).

To see that the vector wavenumber $ \underline{k}= k\,\underline{u}$ has the claimed properties, consider that the orthogonal projection of any vector $ \underline{x}$ onto a vector collinear with $ \underline{u}$ is given by $ (\underline{u}^T\underline{x})\underline{u}$ [451].B.35Thus, $ (\underline{u}^T\underline{x})\underline{u}$ is the component of $ \underline{x}$ lying along the direction of wave propagation indicated by $ \underline{u}$. The norm of this component is $ \vert\vert\,(\underline{u}^T\underline{x})\underline{u}\,\vert\vert =\vert\underline{u}^T\underline{x}\vert$, since $ \underline{u}$ is unit-norm by construction. More generally, $ \underline{u}^T\underline{x}$ is the signed length (in meters) of the component of $ \underline{x}$ along $ \underline{u}$. This length times wavenumber $ k$ gives the spatial phase advance along the wave, or, $ \theta(\underline{x})=k\cdot(\underline{u}^T\underline{x}) \isdeftext \underline{k}^T\underline{x}$.

For another point of view, consider the plane wave $ \cos(\underline{k}^T\underline{x})$, which is the varying portion of the general plane-wave of Eq.$ \,$(B.48) at time $ t=0$, with unit amplitude $ A=1$ and zero phase $ \phi=0$. The spatial phase of this plane wave is given by

$\displaystyle \theta(\underline{x}) \isdefs \underline{k}^T\underline{x}\eqsp k_x x + k_y y + k_z z. $

Recall that the general equation for a plane in 3D space is

$\displaystyle \alpha x + \beta y + \gamma z =$   constant

where $ \alpha$, $ \beta$, and $ \gamma$ are real constants, and $ x$, $ y$, and $ z$ are 3D spatial coordinates. Thus, the set of all points $ \underline{x}^T=(x,y,z)$ yielding the same value $ \theta(\underline{x})=\theta_0$ define a plane of constant phase $ \theta_0$ in $ {\bf R}^3$.

As we know from elementary vector calculus, the direction of maximum phase advance is given by the gradient of the phase $ \theta(\underline{x})$:

$\displaystyle \underline{\nabla }\theta(\underline{x}) \isdefs \left[\begin{ar... ...rray}{c} k_x \\ [2pt] k_y \\ [2pt] k_z\end{array}\right] \isdefs \underline{k} $

This shows that the vector wavenumber $ \underline{k}$ is equal to the gradient of the phase $ \theta(\underline{x})$, so that $ \underline{k}$ points in the direction of maximum spatial-phase advance.

Since the wavenumber $ k$ is the spatial frequency (in radians per meter) along the direction of travel, we should be able to compute it as the directional derivative of $ \theta(\underline{x})$ along $ \underline{k}$, i.e.,

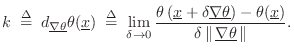
$\displaystyle k \isdefs d_{\underline{\nabla \theta}}\theta(\underline{x}) \isd... ...ta(\underline{x})}{\delta \left\Vert\,\underline{\nabla \theta}\,\right\Vert}. $

An explicit calculation yields

$\displaystyle k \eqsp \left\Vert\,\underline{\nabla \theta}\,\right\Vert \eqsp \sqrt{k_x^2+k_y^2+k_z^2} \isdefs \left\Vert\,\underline{k}\,\right\Vert $

as needed.

Scattering of plane waves is discussed in §C.8.1.

Next Section:
Solving the 2D Wave Equation
Previous Section:
Plane Waves in Air