DSPRelated.com
Free Books
   Free Books    Introduction to Digital Filters  

Complex Resonator

Normally when we need a resonator, we think immediately of the two-pole resonator. However, there is also a complex one-pole resonator having the transfer function

$\displaystyle H(z) = \frac{g}{1-pz^{-1}} \protect$ (B.6)

where $ p=Re^{j\theta_c}$ is the single complex pole, and $ g$ is a scale factor. In the time domain, the complex one-pole resonator is implemented as

$\displaystyle y(n) = g x(n) + p y(n-1). $

Since $ p$ is complex, the output $ y(n)$ is generally complex even when the input $ x(n)$ is real.

Since the impulse response is the inverse z transform of the transfer function, we can write down the impulse response of the complex one-pole resonator by recognizing Eq.$ \,$(B.6) as the closed-form sum of an infinite geometric series, yielding

$\displaystyle h(n) = u(n) g p^n, $

where, as always, $ u(n)$ denotes the unit step function:

$\displaystyle u(n) \isdef \left\{\begin{array}{ll} 1, & n\geq 0 \\ [5pt] 0, & n<0 \\ \end{array}\right. $

Thus, the impulse response is simply a scale factor $ g$ times the geometric sequence $ p^n$ with the pole $ p$ as its ``term ratio''. In general, $ p^n = R^n e^{j\omega_c nT}$ is a sampled, exponentially decaying sinusoid at radian frequency $ \omega_c=\theta_c/T$. By setting $ p$ somewhere on the unit circle to get

$\displaystyle p \isdef e^{j\omega_c T}, $

we obtain a complex sinusoidal oscillator at radian frequency $ \omega_c$ rad/sec. If we like, we can extract the real and imaginary parts separately to create both a sine-wave and a cosine-wave output:

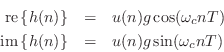
\begin{eqnarray*} \mbox{re}\left\{h(n)\right\} &=& u(n) g \cos(\omega_c n T)\\ \mbox{im}\left\{h(n)\right\} &=& u(n) g \sin(\omega_c n T) \end{eqnarray*}

These may be called phase-quadrature sinusoids, since their phases differ by 90 degrees. The phase quadrature relationship for two sinusoids means that they can be regarded as the real and imaginary parts of a complex sinusoid.

By allowing $ g$ to be complex,

$\displaystyle g \isdef A e^{j\phi} $

we can arbitrarily set both the amplitude and phase of this phase-quadrature oscillator:

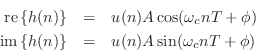
\begin{eqnarray*} \mbox{re}\left\{h(n)\right\} &=& u(n) A \cos(\omega_c n T + \p... ...mbox{im}\left\{h(n)\right\} &=& u(n) A \sin(\omega_c n T + \phi) \end{eqnarray*}

The frequency response of the complex one-pole resonator differs from that of the two-pole real resonator in that the resonance occurs only for one positive or negative frequency $ \omega_c$, but not both. As a result, the resonance frequency $ \omega_c$ is also the frequency where the peak-gain occurs; this is only true in general for the complex one-pole resonator. In particular, the peak gain of a real two-pole filter does not occur exactly at resonance, except when $ \theta_c \isdef \omega_c T = 0$, $ \pi/2$, or $ \pi $. See §B.6 for more on peak-gain versus resonance-gain (and how to normalize them in practice).

Two-Pole Partial Fraction Expansion

Note that every real two-pole resonator can be broken up into a sum of two complex one-pole resonators:

$\displaystyle H(z) = \frac{g}{(1 - p z^{-1}) (1 - \overline{p} z^{-1})} = \frac{g_1}{1-pz^{-1}} + \frac{g_2}{1-\overline{p}z^{-1}} \protect$ (B.7)

where $ g_1$ and $ g_2$ are constants (generally complex). In this ``parallel one-pole'' form, it can be seen that the peak gain is no longer equal to the resonance gain, since each one-pole frequency response is ``tilted'' near resonance by being summed with the ``skirt'' of the other one-pole resonator, as illustrated in Fig.B.9. This interaction between the positive- and negative-frequency poles is minimized by making the resonance sharper ( $ \left\vert p\right\vert\to1$), and by separating the pole frequencies $ 0\ll\angle p \ll \pi$. The greatest separation occurs when the resonance frequency is at one-fourth the sampling rate ( $ \angle p =\pi/2$). However, low-frequency resonances, which are by far the most common in audio work, suffer from significant overlapping of the positive- and negative-frequency poles.

Figure B.9: Frequency response (solid lines) of the two-pole resonator
$ H(z)=1/(1-2R\cos (\theta _c)z^{-1}+ R^2z^{-2})$,
for $ R=0.8$ and $ \theta _c = \pi /8$, overlaid with the frequency responses (dashed lines) of its positive- and negative-frequency complex one-pole components. Also marked (dashed lines) are the two resonance frequencies; the peak frequencies can be seen to lie slightly outside the resonance frequencies.
\includegraphics[width=\twidth ]{eps/tppfe}

To show Eq.$ \,$(B.7) is always true, let's solve in general for $ g_1$ and $ g_2$ given $ g$ and $ p$. Recombining the right-hand side over a common denominator and equating numerators gives

$\displaystyle g = g_1 - g_1 \overline{p}z^{-1}+ g_2 - g_2 pz^{-1} $

which implies

\begin{eqnarray*} g_1+g_2 &=& g\\ g_1 \overline{p} + g_2 p &=& 0. \end{eqnarray*}

The solution is easily found to be

\begin{eqnarray*} g_1 &=& g \frac{p}{2\mbox{im}\left\{p\right\}}\\ g_2 &=& -g \frac{\overline{p}}{2\mbox{im}\left\{p\right\}} \end{eqnarray*}

where we have assumed im$ \left\{p\right\}\neq 0$, as necessary to have a resonator in the first place.

Breaking up the two-pole real resonator into a parallel sum of two complex one-pole resonators is a simple example of a partial fraction expansion (PFE) (discussed more fully in §6.8).

Note that the inverse z transform of a sum of one-pole transfer functions can be easily written down by inspection. In particular, the impulse response of the PFE of the two-pole resonator (see Eq.$ \,$(B.7)) is clearly

$\displaystyle h(n) = g_1 p^n + g_2 \overline{p}^n,\qquad n=0,1,2,\ldots $

Since $ h(n)$ is real, we must have $ g_2=\overline{g}_1$, as we found above without assuming it. If $ \left\vert p\right\vert=1$, then $ h(n)$ is a real sinusoid created by the sum of two complex sinusoids spinning in opposite directions on the unit circle.
Next Section:
The BiQuad Section
Previous Section:
Two-Zero