DSPRelated.com
Free Books
   Free Books    Introduction to Digital Filters  

Impulse Response of Repeated Poles

In the time domain, repeated poles give rise to polynomial amplitude envelopes on the decaying exponentials corresponding to the (stable) poles. For example, in the case of a single pole repeated twice, we have

$\displaystyle \zbox {\frac{1}{\left(1-pz^{-1}\right)^2} \;\longleftrightarrow\; (n+1) p^n, \quad n=0,1,2,\ldots\,.} $


Proof: First note that

$\displaystyle \frac{d}{dz^{-1}}\left(\frac{1}{1-pz^{-1}}\right) = (-1)(1-pz^{-1})^{-2}(-p) = \frac{p}{\left(1-pz^{-1}\right)^2}\;. $

Therefore,
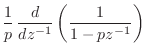$\displaystyle \frac{1}{\left(1-pz^{-1}\right)^2}$ $\displaystyle =$ $\displaystyle \frac{1}{p}\, \frac{d}{dz^{-1}}\left(\frac{1}{1-pz^{-1}}\right)$  
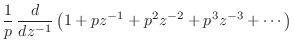  $\displaystyle =$ $\displaystyle \frac{1}{p}\, \frac{d}{dz^{-1}} \left(1 + pz^{-1}+ p^2z^{-2}+ p^3 z^{-3} + \cdots \right)$  
  $\displaystyle =$ $\displaystyle \frac{1}{p} \left(0 + p + 2p^2z^{-1}+ 3p^3z^{-2}+ \cdots \right)$  
  $\displaystyle =$ $\displaystyle 1 + 2pz^{-1}+ 3p^2z^{-2}+ \cdots$  
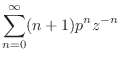  $\displaystyle =$ $\displaystyle \sum_{n=0}^{\infty}(n+1)p^n z^{-n}$  
  $\displaystyle \isdef$ $\displaystyle {\cal Z}\left\{(n+1)p^n\right\} \;\longleftrightarrow\; (n+1)p^n.$ (7.13)

Note that $ n+1$ is a first-order polynomial in $ n$. Similarly, a pole repeated three times corresponds to an impulse-response component that is an exponential decay multiplied by a quadratic polynomial in $ n$, and so on. As long as $ \vert p\vert<1$, the impulse response will eventually decay to zero, because exponential decay always overtakes polynomial growth in the limit as $ n$ goes to infinity.

Next Section:
So What's Up with Repeated Poles?
Previous Section:
Example