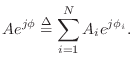DSPRelated.com
Free Books
   Free Books    Introduction to Digital Filters  

Proof Using Complex Variables

To show by means of phasor analysis that Eq.$ \,$(A.2) always has a solution, we can express each component sinusoid as

$\displaystyle A_i\cos(\omega t + \phi_i) =$   re$\displaystyle \left\{A_i e^{j(\omega t + \phi_i)}\right\} $

Equation (A.2) therefore becomes

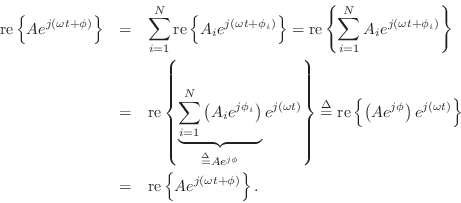
\begin{eqnarray*} \mbox{re}\left\{A e^{j(\omega t + \phi)}\right\} &=& \sum_{i=1... ...}\right\}\\ &=& \mbox{re}\left\{A e^{j(\omega t+\phi)}\right\}. \end{eqnarray*}

Thus, equality holds when we define

$\displaystyle A e^{j\phi} \isdef \sum_{i=1}^N A_i e^{j\phi_i}. \protect$ (A.5)

Since $ A e^{j\phi}$ is just the polar representation of a complex number, there is always some value of $ A\geq 0$ and $ \phi\in[-\pi,\pi)$ such that $ A e^{j\phi}$ equals whatever complex number results on the right-hand side of Eq.$ \,$(A.5).

As is often the case, we see that the use of Euler's identity and complex analysis gives a simplified algebraic proof which replaces a proof based on trigonometric identities.

Next Section:
Phasor Analysis: Factoring a Complex Sinusoid into Phasor Times Carrier
Previous Section:
Proof Using Trigonometry