Probability Distribution
Definition:
A probability distribution
may be defined as a
non-negative real function of all possible outcomes of some random
event. The sum of the probabilities of all possible outcomes is
defined as 1, and probabilities can never be negative.
Example:
A coin toss has two outcomes, ``heads'' (H) or ``tails'' (T),
which are equally likely if the coin is ``fair''. In this case, the
probability distribution is
 |
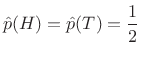(C.1) |
where
denotes the probability of outcome 
. That
is, the total ``probability mass'' is divided equally between the two
possible outcomes heads or tails. This is an example of a
discrete probability distribution because all probability is
assigned to two discrete points, as opposed to
some continuum of possibilities.
Next Section:
Independent Events
Previous Section:
Time-Bandwidth Products Unbounded Above
