DSPRelated.com
Free Books
   Free Books    Introduction to Digital Filters  

Minimum-Phase Polynomials

A filter is minimum phase if both the numerator and denominator of its transfer function are minimum-phase polynomials in $ z^{-1}$:

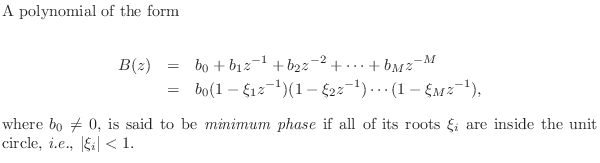
$\textstyle \parbox{0.8\textwidth}{A polynomial of the form \par\begin{center}\b... ...$\ are inside the unit circle, \textit{i.e.}, $\left\vert\xi_i\right\vert<1$. }$
The case $ b_0=0$ is excluded because the polynomial cannot be minimum phase in that case, because then it would have a zero at $ z=\infty$ unless all its coefficients were zero.

As usual, definitions for filters generalize to definitions for signals by simply treating the signal as an impulse response:

$\textstyle \parbox{0.8\textwidth}{A signal $h(n)$, $n\in{\bf Z}$, is said to be minimum phase if its {\it z} transform\ $H(z)$\ is minimum phase. }$

Note that every stable all-pole filter $ H(z)=b_0/A(z)$ is minimum phase, because stability implies that $ A(z)$ is minimum phase, and there are ``no zeros'' (all are at $ z=0$). Thus, minimum phase is the only phase available to a stable all-pole filter.

The contribution of minimum-phase zeros to the complex cepstrum was described in §8.8.

Next Section:
Maximum Phase Filters
Previous Section:
Definition of Minimum Phase Filters