DSPRelated.com
Free Books
   Free Books    Physical Audio Signal Processing  

Young's Modulus

Young's modulus can be thought of as the spring constant for solids. Consider an ideal rod (or bar) of length $ L$ and cross-sectional area $ S$. Suppose we apply a force $ F$ to the face of area $ S$, causing a displacement $ \Delta L$ along the axis of the rod. Then Young's modulus $ Y$ is given by

$\displaystyle Y \isdefs \frac{\mbox{Stress}}{\mbox{Strain}} \isdefs \frac{F/S}{\Delta L/L} $

where

\begin{eqnarray*} F &=& \mbox{total applied force}\\ S &=& \mbox{area over whic... ...a L/L &=& \mbox{\emph{strain} = displacement per unit length}\\ \end{eqnarray*}

For wood, Young's modulus $ Y$ is on the order of $ 10$ N/m$ \null^2$. For aluminum, it is around $ 70$ (a bit higher than glass which is near $ 65$), and structural steel has $ Y\approx 200$ [180].

Young's Modulus as a Spring Constant

Recall (§B.1.3) that Hooke's Law defines a spring constant $ k$ as the applied force $ F$ divided by the spring displacement $ x$, or $ F = k x$. An elastic solid can be viewed as a bundle of ideal springs. Consider, for example, an ideal bar (a rectangular solid in which one dimension, usually its longest, is designated its length $ L$), and consider compression by $ \Delta L$ along the length dimension. The length of each spring in the bundle is the length of the bar, so that each spring constant $ k$ must be inversely proportional to $ L$; in particular, each doubling of length $ L$ doubles the length of each ``spring'' in the bundle, and therefore halves its stiffness. As a result, it is useful to normalize displacement $ \Delta L$ by length $ L$ and use relative displacement $ \Delta L/L$. We need displacement per unit length because we have a constant spring compliance per unit length.

The number of springs in parallel is proportional to the cross-sectional area $ S$ of the bar. Therefore, the force applied to each spring is proportional to the total applied force $ F$ divided by the cross-sectional area $ S$. Thus, Hooke's law for each spring in the bundle can be written

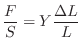
$\displaystyle \frac{F}{S} = Y \frac{\Delta L}{L} $

where $ Y$ is Young's modulus.

We may say that Young's modulus is the Hooke's-law spring constant for the spring made from a specifically cut section of the solid material, cut to length 1 and cross-sectional area 1. The shape of the cross-sectional area does not matter since all displacement is assumed to be longitudinal in this model.

Next Section:
String Tension
Previous Section:
Equations of Motion for Rigid Bodies