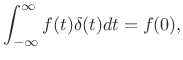DSPRelated.com
Free Books
   Free Books    Spectral Audio Signal Processing  

The Continuous-Time Impulse

An impulse in continuous time must have ``zero width'' and unit area under it. One definition is

$\displaystyle \delta(t) \isdef \lim_{\Delta \to 0} \left\{\begin{array}{ll} \frac{1}{\Delta}, & 0\leq t\leq \Delta \\ [5pt] 0, & \hbox{otherwise}. \\ \end{array} \right. \protect$ (B.26)

An impulse can be similarly defined as the limit of any pulse shape which maintains unit area and approaches zero width at time 0 [150]. As a result, the impulse under every definition has the so-called sifting property under integration,

$\displaystyle \int_{-\infty}^\infty f(t) \delta(t) dt = f(0), \protect$ (B.27)

provided $ f(t)$ is continuous at $ t=0$ . This is often taken as the defining property of an impulse, allowing it to be defined in terms of non-vanishing function limits such as

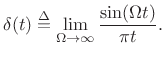$\displaystyle \delta(t) \isdef \lim_{\Omega\to\infty}\frac{\sin(\Omega t)}{\pi t}.$ (B.28)

(Note, incidentally, that $ \sin(\Omega t)/\pi t$ is in $ L2$ but not $ L1$ .)

An impulse is not a function in the usual sense, so it is called instead a distribution or generalized function [36,150]. (It is still commonly called a ``delta function'', however, despite the misnomer.)

Next Section:
Gaussian Pulse
Previous Section:
Power Theorem