Dolph-Chebyshev Window Length Computation

Given a prescribed side-lobe ripple-magnitude and main-lobe width $2\omega_c$ , the required window length is given by [155]

$\displaystyle M = 1 + \frac{\cosh^{-1}(1/r)}{\cosh^{-1}[\sec(\omega_c/2)]}.$

(4.52)

For $\omega_c\ll\pi$ (the typical case), the denominator is close to $\omega_c/2$ , and we have

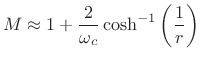$\displaystyle M \approx 1 + \frac{2}{\omega_c}\cosh^{-1}\left(\frac{1}{r}\right)$

(4.53)

Thus, half the time-bandwidth product in radians is approximately

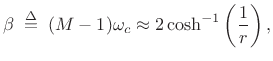$\displaystyle \beta \isdefs (M-1) \omega_c\approx 2\cosh^{-1}\left(\frac{1}{r}\right),$

(4.54)

where $\beta$ is the parameter often used to design Kaiser windows (§3.9).

Next Section:
Convergence of Remez Exchange
Previous Section:
Dolph-Chebyshev Window Main-Lobe Width

Sign in