DSPRelated.com
Free Books
   Free Books    Spectral Audio Signal Processing  

Variance


Definition: The variance or second central moment of a stochastic process $ v(n)$ at time $ n$ is defined as the expected value of $ \left\vert v(n)-\mu_{v(n)}\right\vert^2$ :

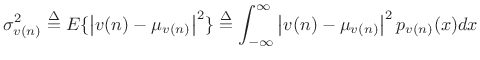$\displaystyle \sigma^2_{v(n)} \isdef E\{\left\vert v(n)-\mu_{v(n)}\right\vert^2\} \isdef \int_{-\infty}^\infty \left\vert v(n)-\mu_{v(n)}\right\vert^2 p_{v(n)}(x) dx$ (C.19)

where $ p_{v(n)}(x)$ is the probability density function for the random variable $ v(n)$ .

For a stationary stochastic process $ v$ , the variance is given by the expected value of $ \left\vert v(n)-\mu_v\right\vert^2$ for any $ n$ .

Next Section:
Sample Variance
Previous Section:
Sample Mean