Laplace Transform Theorems

Free Books Introduction to Digital Filters

Linearity

The Laplace transform is a linear operator. To show this, let denote a linear combination of signals and ,

$\displaystyle w(t) = \alpha x(t) + \beta y(t),$

where $\alpha$ and $\beta$ are real or complex constants. Then we have

$\begin{eqnarray*} W(s) &\isdef & {\cal L}_s\{w\} \isdef {\cal L}_s\{\alpha x(t) ... ..._0^\infty y(t) e^{-st} dt\\ &\isdef & \alpha X(s) + \beta Y(s). \end{eqnarray*}$

Thus, linearity of the Laplace transform follows immediately from the linearity of integration.

Differentiation

The differentiation theorem for Laplace transforms states that

$\displaystyle {\dot x}(t) \leftrightarrow s X(s) - x(0),$

where ${\dot x}(t) \isdef \frac{d}{dt}x(t)$ , and

is any differentiable function that approaches zero as

goes to infinity. In operator notation,

$\displaystyle \zbox {{\cal L}_{s}\{{\dot x}\} = s X(s) - x(0).}$

Proof: This follows immediately from integration by parts:

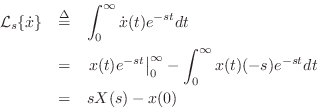
$\begin{eqnarray*} {\cal L}_{s}\{{\dot x}\} &\isdef & \int_{0}^\infty {\dot x}(t)... ...y} - \int_{0}^\infty x(t) (-s)e^{-s t} dt\\ &=& s X(s) - x(0) \end{eqnarray*}$

since $x(\infty)=0$ by assumption.

Corollary: Integration Theorem

$\displaystyle \zbox {{\cal L}_{s}\left\{\int_0^t x(\tau)d\tau\right\} = \frac{X(s)}{s}}$

Thus, successive time derivatives correspond to successively higher powers of , and successive integrals with respect to time correspond to successively higher powers of .

Next Section:
Laplace Analysis of Linear Systems
Previous Section:
Relation to the z Transform