Free Books    Introduction to Digital Filters  

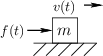
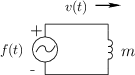
Moving Mass

Figure D.1 depicts a free mass driven by an external force along an ideal frictionless surface in one dimension. Figure D.2 shows the electrical equivalent circuit for this scenario in which the external force is represented by a voltage source emitting $ f(t)$ volts, and the mass is modeled by an inductor having the value $ L=m$ Henrys.

Figure D.1: Physical diagram of an external force driving a mass along a frictionless surface.
\begin{figure}\input fig/forcemass.pstex_t \end{figure}

Figure: Electrical equivalent circuit of the force-driven mass in Fig.D.1.
\begin{figure}\input fig/forcemassec.pstex_t \end{figure}

From Newton's second law of motion ``$ f=ma$'', we have

$\displaystyle f(t) = m\,a(t) \isdef m\,{\dot v}(t) \isdef m\,{\ddot x}(t). $

Taking the unilateral Laplace transform and applying the differentiation theorem twice yields

\begin{eqnarray*} F(s) &=& m\,{\cal L}_s\{{\ddot x}\}\\ &=& m\left[\,s {\cal L... ...right\}\\ &=& m\left[s^2\,X(s) - s\,x(0) - {\dot x}(0)\right]. \end{eqnarray*}

Thus, given

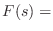  • $ F(s) = $ Laplace transform of the driving force $ f(t)$,
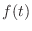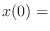  • $ x(0) = $ initial mass position, and
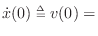  • $ {\dot x}(0)\isdeftext v(0) = $ initial mass velocity,
we can solve algebraically for $ X(s)$, the Laplace transform of the mass position for all $ t\ge 0$. This Laplace transform can then be inverted to obtain the mass position $ x(t)$ for all $ t\ge 0$. This is the general outline of how Laplace-transform analysis goes for all linear, time-invariant (LTI) systems. For nonlinear and/or time-varying systems, Laplace-transform analysis cannot, strictly speaking, be used at all.

If the applied external force $ f(t)$ is zero, then, by linearity of the Laplace transform, so is $ F(s)$, and we readily obtain

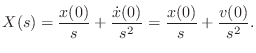
$\displaystyle X(s) = \frac{x(0)}{s} + \frac{{\dot x}(0)}{s^2} = \frac{x(0)}{s} + \frac{v(0)}{s^2}. $

Since $ 1/s$ is the Laplace transform of the Heaviside unit-step function

$\displaystyle u(t)\isdef \left\{\begin{array}{ll} 0, & t<0 \\ [5pt] 1, & t\ge 0 \\ \end{array}\right., $

we find that the position of the mass $ x(t)$ is given for all time by

$\displaystyle x(t) = x(0)\,u(t) + v(0)\,t\,u(t). $

Thus, for example, a nonzero initial position $ x(0)=x_0$ and zero initial velocity $ v(0)=0$ results in $ x(t)=x_0$ for all $ t\ge 0$; that is, the mass ``just sits there''.D.3 Similarly, any initial velocity $ v(0)$ is integrated with respect to time, meaning that the mass moves forever at the initial velocity.

To summarize, this simple example illustrated use the Laplace transform to solve for the motion of a simple physical system (an ideal mass) in response to initial conditions (no external driving forces). The system was described by a differential equation which was converted to an algebraic equation by the Laplace transform.

Next Section:
Mass-Spring Oscillator Analysis
Previous Section:
Differentiation