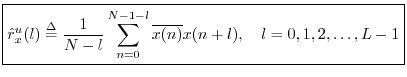Free Books    Mathematics of the DFT  

Autocorrelation

The cross-correlation of a signal with itself gives its autocorrelation:

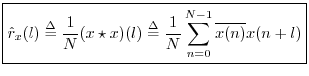
$\displaystyle \zbox {{\hat r}_x(l) \isdef \frac{1}{N}(x\star x)(l) \isdef \frac{1}{N}\sum_{n=0}^{N-1}\overline{x(n)} x(n+l)} $

The autocorrelation function is Hermitian:

$\displaystyle {\hat r}_x(-l) = \overline{{\hat r}_x(l)} $

When $ x$ is real, its autocorrelation is real and even (symmetric about lag zero).

The unbiased cross-correlation similarly reduces to an unbiased autocorrelation when $ x\equiv y$:

$\displaystyle \zbox {{\hat r}^u_x(l) \isdef \frac{1}{N-l}\sum_{n=0}^{N-1-l} \overline{x(n)} x(n+l),\quad l = 0,1,2,\ldots,L-1} \protect$ (8.2)

The DFT of the true autocorrelation function $ r_x(n)\in{\bf R}^N$ is the (sampled) power spectral density (PSD), or power spectrum, and may be denoted

$\displaystyle R_x(\omega_k) \isdef \hbox{\sc DFT}_k(r_x). $

The complete (not sampled) PSD is $ R_x(\omega) \isdef \hbox{\sc DTFT}_k(r_x)$, where the DTFT is defined in Appendix B (it's just an infinitely long DFT). The DFT of $ {\hat r}_x$ thus provides a sample-based estimate of the PSD:8.10

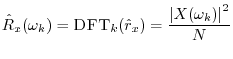
$\displaystyle {\hat R}_x(\omega_k)=\hbox{\sc DFT}_k({\hat r}_x) = \frac{\left\vert X(\omega_k)\right\vert^2}{N} $

We could call $ {\hat R}_x(\omega_k)$ a ``sampled sample power spectral density''.

At lag zero, the autocorrelation function reduces to the average power (mean square) which we defined in §5.8:

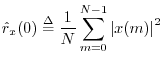
$\displaystyle {\hat r}_x(0) \isdef \frac{1}{N}\sum_{m=0}^{N-1}\left\vert x(m)\right\vert^2 % \isdef \Pscr_x^2 $

Replacing ``correlation'' with ``covariance'' in the above definitions gives corresponding zero-mean versions. For example, we may define the sample circular cross-covariance as

$\displaystyle \zbox {{\hat c}_{xy}(n) \isdef \frac{1}{N}\sum_{m=0}^{N-1}\overline{[x(m)-\mu_x]} [y(m+n)-\mu_y].} $

where $ \mu_x$ and $ \mu_y$ denote the means of $ x$ and $ y$, respectively. We also have that $ {\hat c}_x(0)$ equals the sample variance of the signal $ x$:

$\displaystyle {\hat c}_x(0) \isdef \frac{1}{N}\sum_{m=0}^{N-1}\left\vert x(m)-\mu_x\right\vert^2 \isdef {\hat \sigma}_x^2 $

Next Section:
Matched Filtering
Previous Section:
Unbiased Cross-Correlation