Free Books    Mathematics of the DFT  

e^(j theta)

We've now defined $ a^z$ for any positive real number $ a$ and any complex number $ z$. Setting $ a=e$ and $ z=j\theta$ gives us the special case we need for Euler's identity. Since $ e^x$ is its own derivative, the Taylor series expansion for $ f(x)=e^x$ is one of the simplest imaginable infinite series:

$\displaystyle e^x = \sum_{n=0}^\infty \frac{x^n}{n!} = 1 + x + \frac{x^2}{2} + \frac{x^3}{3!} + \cdots $

The simplicity comes about because $ f^{(n)}(0)=1$ for all $ n$ and because we chose to expand about the point $ x=0$. We of course define

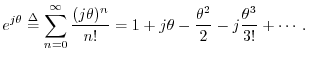
$\displaystyle e^{j\theta} \isdef \sum_{n=0}^\infty \frac{(j\theta)^n}{n!} = 1 + j\theta - \frac{\theta^2}{2} - j\frac{\theta^3}{3!} + \cdots \,. $

Note that all even order terms are real while all odd order terms are imaginary. Separating out the real and imaginary parts gives

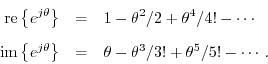
\begin{eqnarray*} \mbox{re}\left\{e^{j\theta}\right\} &=& 1 - \theta^2/2 + \thet... ...heta}\right\} &=& \theta - \theta^3/3! + \theta^5/5! - \cdots\,. \end{eqnarray*}

Comparing the Maclaurin expansion for $ e^{j\theta }$ with that of $ \cos(\theta)$ and $ \sin(\theta)$ proves Euler's identity. Recall from introductory calculus that

\begin{eqnarray*} \frac{d}{d\theta}\cos(\theta) &=& -\sin(\theta) \\ [5pt] \frac{d}{d\theta}\sin(\theta) &=& \cos(\theta) \end{eqnarray*}

so that

\begin{eqnarray*} \left.\frac{d^n}{d\theta^n}\cos(\theta)\right\vert _{\theta=0}... ...} \\ [5pt] 0, & n\;\mbox{\small even}. \\ \end{array} \right. \end{eqnarray*}

Plugging into the general Maclaurin series gives

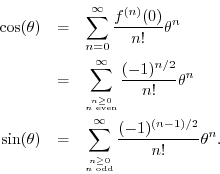
\begin{eqnarray*} \cos(\theta) &=& \sum_{n=0}^\infty \frac{f^{(n)}(0)}{n!}\theta... ...mbox{\tiny$n$\ odd}}}^\infty \frac{(-1)^{(n-1)/2}}{n!} \theta^n. \end{eqnarray*}

Separating the Maclaurin expansion for $ e^{j\theta }$ into its even and odd terms (real and imaginary parts) gives

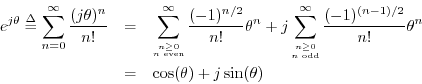
\begin{eqnarray*} e^{j\theta} \isdef \sum_{n=0}^\infty \frac{(j\theta)^n}{n!} &... ...-1)^{(n-1)/2}}{n!} \theta^n\\ &=& \cos(\theta) + j\sin(\theta) \end{eqnarray*}

thus proving Euler's identity.

Next Section:
Back to Mth Roots
Previous Section:
Back to e