DSPRelated.com
Free Books
   Free Books    Physical Audio Signal Processing  

The Ideal Vibrating String

Figure C.1: The ideal vibrating string.
\includegraphics[width=\twidth]{eps/Fphysicalstring}

The wave equation for the ideal (lossless, linear, flexible) vibrating string, depicted in Fig.C.1, is given by

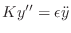$\displaystyle Ky''= \epsilon {\ddot y}$ (C.1)

where

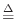
\begin{displaymath}\begin{array}{rclrcl} K& \isdef & \mbox{string tension} & \qq... ...isdef & \frac{\partial}{\partial x}y(t,x) \nonumber \end{array}\end{displaymath}    

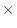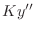
where ``$ \isdef $'' means ``is defined as.'' The wave equation is derived in §B.6. It can be interpreted as a statement of Newton's second law, ``force = mass $ \times$ acceleration,'' on a microscopic scale. 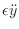Since we are concerned with transverse vibrations on the string, the relevant restoring force (per unit length) is given by the string tension times the curvature of the string ($ Ky''$); the restoring force is balanced at all times by the inertial force per unit length of the string which is equal to mass density times transverse acceleration ( $ \epsilon {\ddot y}$).

Next Section:
The Finite Difference Approximation
Previous Section:
Wave Equation in Higher Dimensions