Duality of COLA and Nyquist Conditions

Free Books Spectral Audio Signal Processing

Let $\hbox{\sc Cola}(N)$ denote constant overlap-add using hop size . Then we have (by the Poisson summation formula Eq. $\,$ (8.30))

$\begin{eqnarray*} w &\in& \hbox{\sc Nyquist}(N) \Leftrightarrow W \in \hbox{\sc Cola}(2\pi/N) \qquad \hbox{(FBS)} \\ [10pt] w &\in& \hbox{\sc Cola}(R) \Leftrightarrow W \in \hbox{\sc Nyquist}(2\pi/R) \qquad \hbox{(OLA)} \end{eqnarray*}$

Specific Windows

Recall that the rectangular window transform is $\hbox{\sc Nyquist}(2\pi/M)$ , implying the rectangular window itself is $\hbox{\sc Cola}(M)$ , which is obvious.
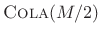The window transform for the Hamming family is $\hbox{\sc Nyquist}(4\pi/M)$ , implying that Hamming windows are $\hbox{\sc Cola}(M/2)$ , which we also knew.
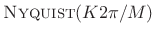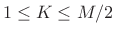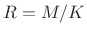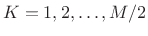The rectangular window transform is also $\hbox{\sc Nyquist}(K2\pi/M)$ for any integer $1\leq K\leq M/2$ , implying that all hop sizes given by for $K=1,2,\ldots,M/2$ are COLA.
Because its side lobes are the same width as the sinc side lobes, the Hamming window transform is also 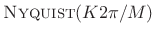$\hbox{\sc Nyquist}(K2\pi/M)$ ,for any integer $2\leq K\leq M/2$ , implying hop sizes 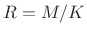are good, for 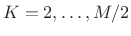$K=2,\ldots,M/2$ . Thus, the available hop sizes for the Hamming window family include all of those for the rectangular window except one ( ).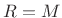

The Nyquist Property on the Unit Circle

As a degenerate case, note that is COLA for any window, while no window transform is $\hbox{\sc Nyquist}(2\pi)$ except the zero window. (since it would have to be zero at dc, and we do not consider such windows). Did the theory break down for ?

Intuitively, the $\hbox{\sc Nyquist}(2\pi/R)$ condition on the window transform $W(\omega)$ ensures that all nonzero multiples of the time-domain-frame-rate $2\pi/R$ will be zeroed out over the interval $[-\pi,\pi)$ along the frequency axis. When the frame-rate equals the sampling rate ( ), there are no frame-rate multiples in the range $[-\pi,\pi)$ . (The range $[0,2\pi)$ gives the same result.) When , there is exactly one frame-rate multiple at $-\pi$ . When , there are two at $\pm 2\pi/3$ . When , they are at $-\pi$ and $\pm\pi/2$ , and so on.

We can cleanly handle the special case of by defining all functions over the unit circle as being $\hbox{\sc Nyquist}(2\pi)$ when there are no frame-rate multiples in the range $[-\pi,\pi)$ . Thus, a discrete-time spectrum $W(\omega), \omega\in[-\pi,\pi)$ is said to be $\hbox{\sc Nyquist}(2\pi/K)$ if $W(r 2\pi/K)=0$ , for all $\vert r\vert=1,2,\ldots,\left\lfloor K/2\right\rfloor$ , where $\left\lfloor x\right\rfloor$ (the ``floor function'') denotes the greatest integer less than or equal to .