DSPRelated.com
Free Books
   Free Books    Spectral Audio Signal Processing  

Sample Power Spectral Density

The Fourier transform of the sample autocorrelation function $ \hat{r}_{v,N}$ (see (6.6)) is defined as the sample power spectral density (PSD):

$\displaystyle {\hat S}_{v,N}(\omega) \isdef \hbox{\sc DTFT}_\omega\{\hat{r}_{v,N}\} \isdef \sum_{n=-\infty}^\infty \hat{r}_{v,N}(n)e^{-j\omega n}$ (7.11)

This definition coincides with the classical periodogram when $ \hat{r}_{v,N}$ is weighted differently (by a Bartlett window).

Similarly, the true power spectral density of a stationary stochastic processes $ v(n)$ is given by the Fourier transform of the true autocorrelation function $ r_v(l)$ , i.e.,

$\displaystyle S_{v}(\omega) = \hbox{\sc DTFT}_\omega\{r_v\}.$ (7.12)

For real signals, the autocorrelation function is always real and even, and therefore the power spectral density is real and even for all real signals. An area under the PSD, $ S_x(\omega)\cdot\Delta\omega$ , comprises the contribution to the variance of $ x(n)$ from the frequency interval $ [\omega,\omega+\Delta\omega]$ . The total integral of the PSD gives the total variance:

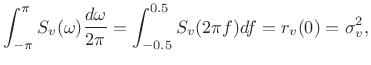$\displaystyle \int_{-\pi}^\pi S_v(\omega) \frac{d\omega}{2\pi} = \int_{-0.5}^{0.5} S_v(2\pi f) df = r_v(0) = \sigma_v^2,$ (7.13)

again assuming $ v(n)$ is zero mean.7.5

Since the sample autocorrelation of white noise approaches an impulse, its PSD approaches a constant, as can be seen in Fig.6.1. This means that white noise contains all frequencies in equal amounts. Since white light is defined as light of all colors in equal amounts, the term ``white noise'' is seen to be analogous.

Next Section:
Biased Sample Autocorrelation
Previous Section:
Sample Autocorrelation