Free Books    Mathematics of the DFT  

Sinusoidal Amplitude Modulation (AM)

It is instructive to study the modulation of one sinusoid by another. In this section, we will look at sinusoidal Amplitude Modulation (AM). The general AM formula is given by

$\displaystyle x_\alpha(t) = [1+\alpha \cdot a_m(t)]\cdot A_c\sin(\omega_c t + \phi_c), $

where $ (A_c,\omega_c,\phi_c)$ are parameters of the sinusoidal carrier wave, $ \alpha\in[0,1]$ is called the modulation index (or AM index), and $ a_m(t)\in[-1,1]$ is the amplitude modulation signal. In AM radio broadcasts, $ a_m(t)$ is the audio signal being transmitted (usually bandlimited to less than 10 kHz), and $ \omega_c$ is the channel center frequency that one dials up on a radio receiver. The modulated signal $ x_\alpha(t)$ can be written as the sum of the unmodulated carrier wave plus the product of the carrier wave and the modulating wave:

$\displaystyle x_\alpha(t) = x_0(t) + \alpha \cdot a_m(t) \cdot A_c\sin(\omega_c t + \phi_c) \protect$ (4.1)

In the case of sinusoidal AM, we have

$\displaystyle a_m(t) = \sin(\omega_m t + \phi_m). \protect$ (4.2)

Periodic amplitude modulation of this nature is often called the tremolo effect when $ \omega_m<20\pi$ or so ($ <10$ Hz).

Let's analyze the second term of Eq.$ \,$(4.1) for the case of sinusoidal AM with $ \alpha =1$ and $ \phi_m=\phi_c=0$:

$\displaystyle x_m(t) \isdef \sin(\omega_m t)\sin(\omega_c t) \protect$ (4.3)

An example waveform is shown in Fig.4.11 for $ f_c=100$ Hz and $ f_m=10$ Hz. Such a signal may be produced on an analog synthesizer by feeding two differently tuned sinusoids to a ring modulator, which is simply a ``four-quadrant multiplier'' for analog signals.

Figure: Sinusoidal amplitude modulation as in Eq.$ \,$(4.3)--time waveform.
\includegraphics[width=3.5in]{eps/sineamtd}

When $ \omega_m$ is small (say less than $ 20\pi$ radians per second, or 10 Hz), the signal $ x_m(t)$ is heard as a ``beating sine wave'' with $ \omega_m/\pi=2f_m$ beats per second. The beat rate is twice the modulation frequency because both the positive and negative peaks of the modulating sinusoid cause an ``amplitude swell'' in $ x_m(t)$. (One period of modulation--$ 1/f_m$ seconds--is shown in Fig.4.11.) The sign inversion during the negative peaks is not normally audible.

Recall the trigonometric identity for a sum of angles:

$\displaystyle \cos(A+B) = \cos(A)\cos(B) - \sin(A)\sin(B) $

Subtracting this from $ \cos(A-B) = \cos(A)\cos(B) + \sin(A)\sin(B)$ leads to the identity

$\displaystyle \sin(A)\sin(B) = \frac{\cos(A-B) - \cos(A+B)}{2}. $

Setting $ A=\omega_m t$ and $ B=\omega_c t$ gives us an alternate form for our ``ring-modulator output signal'':

$\displaystyle x_m(t) \isdef \sin(\omega_m t)\sin(\omega_c t) = \frac{\cos[(\omega_m-\omega_c)t] - \cos[(\omega_m+\omega_c)t]}{2} \protect$ (4.4)

These two sinusoidal components at the sum and difference frequencies of the modulator and carrier are called side bands of the carrier wave at frequency $ \omega_c$ (since typically $ \omega_c\gg\omega_m>0$).

Equation (4.3) expresses $ x_m(t)$ as a ``beating sinusoid'', while Eq.$ \,$(4.4) expresses as it two unmodulated sinusoids at frequencies $ \omega_c\pm\omega_m$. Which case do we hear?

It turns out we hear $ x_m(t)$ as two separate tones (Eq.$ \,$(4.4)) whenever the side bands are resolved by the ear. As mentioned in §4.1.2, the ear performs a ``short time Fourier analysis'' of incoming sound (the basilar membrane in the cochlea acts as a mechanical filter bank). The resolution of this filterbank--its ability to discern two separate spectral peaks for two sinusoids closely spaced in frequency--is determined by the critical bandwidth of hearing [45,76,87]. A critical bandwidth is roughly 15-20% of the band's center-frequency, over most of the audio range [71]. Thus, the side bands in sinusoidal AM are heard as separate tones when they are both in the audio range and separated by at least one critical bandwidth. When they are well inside the same critical band, ``beating'' is heard. In between these extremes, near separation by a critical-band, the sensation is often described as ``roughness'' [29].

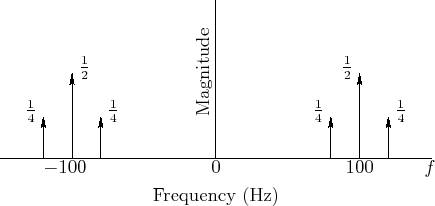
Example AM Spectra

Equation (4.4) can be used to write down the spectral representation of $ x_m(t)$ by inspection, as shown in Fig.4.12. In the example of Fig.4.12, we have $ f_c=100$ Hz and $ f_m=20$ Hz, where, as always, $ \omega=2\pi f$. For comparison, the spectral magnitude of an unmodulated $ 100$ Hz sinusoid is shown in Fig.4.6. Note in Fig.4.12 how each of the two sinusoidal components at $ \pm100$ Hz have been ``split'' into two ``side bands'', one $ 20$ Hz higher and the other $ 20$ Hz lower, that is, $ \pm100\pm20=\{-120,-80,80,120\}$. Note also how the amplitude of the split component is divided equally among its two side bands.

figure[htbp] \includegraphics{eps/sineamfd}

Recall that $ x_m(t)$ was defined as the second term of Eq.$ \,$(4.1). The first term is simply the original unmodulated signal. Therefore, we have effectively been considering AM with a ``very large'' modulation index. In the more general case of Eq.$ \,$(4.1) with $ a_m(t)$ given by Eq.$ \,$(4.2), the magnitude of the spectral representation appears as shown in Fig.4.13.

figure[htbp] \includegraphics{eps/sineamgfd}

Next Section:
Sinusoidal Frequency Modulation (FM)
Previous Section:
Plotting Complex Sinusoids versus Frequency