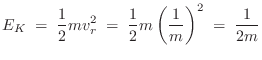Two Masses Connected by a Rod
As an introduction to the decomposition of rigid-body motion into
translational and rotational components, consider the
simple system shown in Fig.B.5. The excitation force
densityB.15 ![]() can be
applied anywhere between
can be
applied anywhere between ![]() and
and ![]() along the connecting rod.
We will deliver a vertical impulse of momentum to the mass on the
right, and show, among other observations, that the total kinetic
energy is split equally into (1) the rotational kinetic energy
about the center of mass, and (2) the translational kinetic
energy of the total mass, treated as being located at the center of
mass. This is accomplished by defining a new frame of
reference (i.e., a moving coordinate system) that has its origin at
the center of mass.
along the connecting rod.
We will deliver a vertical impulse of momentum to the mass on the
right, and show, among other observations, that the total kinetic
energy is split equally into (1) the rotational kinetic energy
about the center of mass, and (2) the translational kinetic
energy of the total mass, treated as being located at the center of
mass. This is accomplished by defining a new frame of
reference (i.e., a moving coordinate system) that has its origin at
the center of mass.
First, note that the driving-point impedance (§7.1)
``seen'' by the driving force ![]() varies as a function of
varies as a function of ![]() .
At
.
At ![]() , The excitation
, The excitation ![]() sees a ``point mass''
sees a ``point mass'' ![]() , and no
rotation is excited by the force (by symmetry). At
, and no
rotation is excited by the force (by symmetry). At ![]() , on the
other hand, the excitation
, on the
other hand, the excitation
![]() only sees mass
only sees mass ![]() at time
0, because the vertical motion of either point-mass initially only
rotates the other point-mass via the massless connecting rod. Thus,
an observation we can make right away is that the driving point
impedance seen by
at time
0, because the vertical motion of either point-mass initially only
rotates the other point-mass via the massless connecting rod. Thus,
an observation we can make right away is that the driving point
impedance seen by ![]() depends on the striking point
depends on the striking point ![]() and,
away from
and,
away from ![]() , it depends on time
, it depends on time ![]() as well.
as well.
To avoid dealing with a time-varying driving-point impedance, we will
use an impulsive force input at time ![]() . Since momentum is the
time-integral of force (
. Since momentum is the
time-integral of force (
![]() ), our
excitation will be a unit momentum transferred to the two-mass
system at time 0.
), our
excitation will be a unit momentum transferred to the two-mass
system at time 0.
Striking the Rod in the Middle
First, consider
![]() . That is, we apply an
upward unit-force impulse at time 0 in the middle of the rod. The
total momentum delivered in the neighborhood of
. That is, we apply an
upward unit-force impulse at time 0 in the middle of the rod. The
total momentum delivered in the neighborhood of ![]() and
and ![]() is
obtained by integrating the applied force density with respect to time
and position:
is
obtained by integrating the applied force density with respect to time
and position:
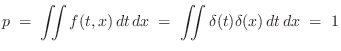
The kinetic energy of the system after time zero is
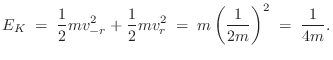

Striking One of the Masses
Now let
![]() . That is, we apply an impulse
of vertical momentum to the mass on the right at time 0.
. That is, we apply an impulse
of vertical momentum to the mass on the right at time 0.
In this case, the unit of vertical momentum is transferred entirely to the mass on the right, so that
Note that the velocity of the center-of-mass ![]() is the
same as it was when we hit the midpoint of the rod. This is an
important general equivalence: The sum of all external force vectors
acting on a rigid body can be applied as a single resultant force
vector to the total mass concentrated at the center of mass to find
the linear (translational) motion produced. (Recall from §B.4.1
that such a sum is the same as the sum of all radially acting external
force components, since the tangential components contribute only to
rotation and not to translation.)
is the
same as it was when we hit the midpoint of the rod. This is an
important general equivalence: The sum of all external force vectors
acting on a rigid body can be applied as a single resultant force
vector to the total mass concentrated at the center of mass to find
the linear (translational) motion produced. (Recall from §B.4.1
that such a sum is the same as the sum of all radially acting external
force components, since the tangential components contribute only to
rotation and not to translation.)
All of the kinetic energy is in the mass on the right just after time zero:
However, after time zero, things get more complicated, because the mass on the left gets dragged into a rotation about the center of mass.
To simplify ongoing analysis, we can define a body-fixed frame
of referenceB.16 having its origin at the center of mass. Let ![]() denote a velocity in this frame. Since the velocity of the center of
mass is
denote a velocity in this frame. Since the velocity of the center of
mass is
![]() , we can convert any velocity
, we can convert any velocity ![]() in the
body-fixed frame to a velocity
in the
body-fixed frame to a velocity ![]() in the original frame by adding
in the original frame by adding
![]() to it, viz.,
to it, viz.,
In the body-fixed frame, all kinetic energy is rotational about
the origin. Recall (Eq.![]() (B.9)) that the moment of inertia for this
system, with respect to the center of mass at
(B.9)) that the moment of inertia for this
system, with respect to the center of mass at ![]() , is
, is
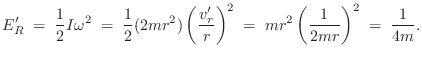
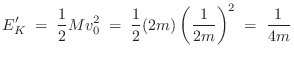
In summary, we defined a moving body-fixed frame having its origin at the center-of-mass, and the total kinetic energy was computed to be
It is important to note that, after time zero, both the linear
momentum of the center-of-mass (
![]() ), and the angular momentum in the body-fixed frame
(
), and the angular momentum in the body-fixed frame
(
![]() ) remain
constant over time.B.17 In the original space-fixed
frame, on the other hand, there is a complex transfer of momentum back
and forth between the masses after time zero.
) remain
constant over time.B.17 In the original space-fixed
frame, on the other hand, there is a complex transfer of momentum back
and forth between the masses after time zero.
Similarly, the translational kinetic energy of the total mass, treated as being concentrated at its center-of-mass, and the rotational kinetic energy in the body-fixed frame, are both constant after time zero, while in the space-fixed frame, kinetic energy transfers back and forth between the two masses. At all times, however, the total kinetic energy is the same in both formulations.
Next Section:
Angular Velocity Vector
Previous Section:
Radius of Gyration








![\includegraphics[width=1.5in]{eps/massrodmass}](http://www.dsprelated.com/josimages_new/pasp/img2758.png)