Free Books    Spectral Audio Signal Processing  

Convolution Theorem for the DTFT

The convolution of discrete-time signals $ x$ and $ y$ is defined as

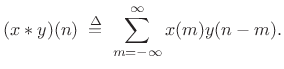$\displaystyle (x \ast y)(n) \isdefs \sum_{m=-\infty}^\infty x(m)y(n-m).$ (3.22)

This is sometimes called acyclic convolution to distinguish it from the cyclic convolution used for length $ N$ sequences in the context of the DFT [264]. Convolution is cyclic in the time domain for the DFT and FS cases (i.e., whenever the time domain has a finite length), and acyclic for the DTFT and FT cases.3.6

The convolution theorem is then

$\displaystyle \zbox {(x\ast y) \;\longleftrightarrow\;X \cdot Y}$ (3.23)

That is, convolution in the time domain corresponds to pointwise multiplication in the frequency domain.


Proof: The result follows immediately from interchanging the order of summations associated with the convolution and DTFT:

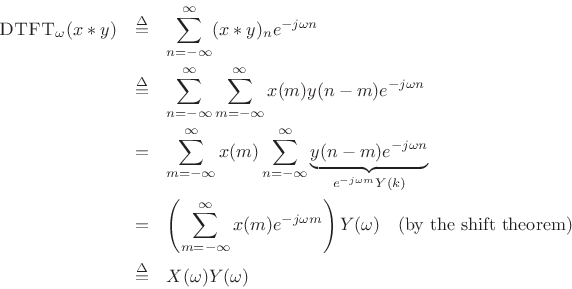
\begin{eqnarray*} \hbox{\sc DTFT}_\omega(x\ast y) &\isdef & \sum_{n=-\infty}^{\infty}(x\ast y)_n e^{-j\omega n} \\ &\isdef & \sum_{n=-\infty}^{\infty}\sum_{m=-\infty}^{\infty}x(m) y(n-m) e^{-j\omega n} \\ &=& \sum_{m=-\infty}^{\infty}x(m) \sum_{n=-\infty}^{\infty}\underbrace{y(n-m) e^{-j\omega n}}_{e^{-j\omega m}Y(k)} \\ &=& \left(\sum_{m=-\infty}^{\infty}x(m) e^{-j\omega m}\right)Y(\omega)\quad\mbox{(by the shift theorem)}\\ &\isdef & X(\omega)Y(\omega) \end{eqnarray*}

Next Section:
Correlation Theorem for the DTFT
Previous Section:
Shift Theorem for the DTFT