DSPRelated.com
Free Books
   Free Books    Physical Audio Signal Processing  

Waveguide Transformers and Gyrators

The ideal transformer, depicted in Fig. C.37 a, is a lossless two-port electric circuit element which scales up voltage by a constant $ g$ [110,35]. In other words, the voltage at port 2 is always $ g$ times the voltage at port 1. Since power is voltage times current, the current at port 2 must be $ 1/g$ times the current at port 1 in order for the transformer to be lossless. The scaling constant $ g$ is called the turns ratio because transformers are built by coiling wire around two sides of a magnetically permeable torus, and the number of winds around the port 2 side divided by the winding count on the port 1 side gives the voltage stepping constant $ g$.

Figure C.37: a) Two-port description of the ideal transformer with ``turns ratio'' $ g$. b) Corresponding wave digital transformer.
\includegraphics[width=\twidth]{eps/lTransformer}

In the case of mechanical circuits, the two-port transformer relations appear as

\begin{eqnarray*} F_2(s) &=& g F_1(s) \\ [5pt] V_2(s) &=& \frac{1}{g} V_1(s) \end{eqnarray*}

where $ F$ and $ V$ denote force and velocity, respectively. We now convert these transformer describing equations to the wave variable formulation. Let $ R_1$ and $ R_2$ denote the wave impedances on the port 1 and port 2 sides, respectively, and define velocity as positive into the transformer. Then

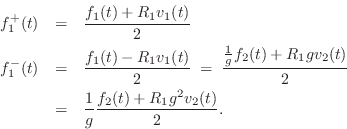
\begin{eqnarray*} f^{{+}}_1(t) &=& \frac{f_1(t) + R_1 v_1(t)}{2} \\ f^{{-}}_1(t... ...2(t)}{2} \\ &=& \frac{1}{g} \frac{f_2(t) + R_1 g^2 v_2(t)}{2}. \end{eqnarray*}

Similarly,

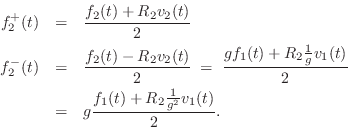
\begin{eqnarray*} f^{{+}}_2(t) &=& \frac{f_2(t) + R_2 v_2(t)}{2} \\ f^{{-}}_2(t... ...1(t)}{2} \\ &=& g \frac{f_1(t) + R_2 \frac{1}{g^2} v_1(t)}{2}. \end{eqnarray*}

We see that choosing

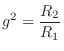
$\displaystyle g^2 = \frac{R_2}{R_1} $

eliminates the scattering terms and gives the simple relations

\begin{eqnarray*} f^{{-}}_2(t) &=& g f^{{+}}_1(t)\\ [5pt] f^{{-}}_1(t) &=& \frac{1}{g}f^{{+}}_2(t). \end{eqnarray*}

The corresponding wave flow diagram is shown in Fig. C.37 b.

Thus, a transformer with a voltage gain $ g$ corresponds to simply changing the wave impedance from $ R_1$ to $ R_2$, where $ g=\sqrt{R_2/R_1}$. Note that the transformer implements a change in wave impedance without scattering as occurs in physical impedance steps (§C.8).

Gyrators

Another way to define the ideal waveguide transformer is to ask for a two-port element that joins two waveguide sections of differing wave impedance in such a way that signal power is preserved and no scattering occurs. From Ohm's Law for traveling waves (Eq.$ \,$(6.6)), and from the definition of power waves (§C.7.5), we see that to bridge an impedance discontinuity between $ R_{i-1}$ and $ R_i$ with no power change and no scattering requires the relations

$\displaystyle \frac{[f^{{+}}_i]^2}{R_i} = \frac{[f^{{+}}_{i-1}]^2}{R_{i-1}}, \qquad\qquad \frac{[f^{{-}}_i]^2}{R_i} = \frac{[f^{{-}}_{i-1}]^2}{R_{i-1}}. $

Therefore, the junction equations for a transformer can be chosen as

$\displaystyle f^{{+}}_i= g_i\, f^{{+}}_{i-1}\qquad\qquad f^{{-}}_{i-1}= g_i^{-1}\, f^{{-}}_i \protect$ (C.125)

where

$\displaystyle g_i \isdef \pm\sqrt{\frac{R_i}{R_{i-1}}} \protect$ (C.126)

Choosing the negative square root for $ g_i^{-1}$ gives a gyrator [35]. Gyrators are often used in electronic circuits to replace inductors with capacitors. The gyrator can be interpreted as a transformer in cascade with a dualizer [433]. A dualizer converts one from wave variable type (such as force) to the other (such as velocity) in the waveguide.

The dualizer is readily derived from Ohm's Law for traveling waves:

\begin{eqnarray*} f^{{+}}\eqsp Rv^{+}, \qquad f^{{-}}\eqsp -Rv^{-}\\ [5pt] \Lon... ...i\eqsp Rv^{+}_{i-1}, \qquad v^{-}_{i-1} \eqsp -R^{-1} f^{{-}}_i \end{eqnarray*}

In this case, velocity waves in section $ i-1$ are converted to force waves in section $ i$, and vice versa (all at wave impedance $ R$). The wave impedance can be changed as well by cascading a transformer with the dualizer, which changes $ R$ to $ R\sqrt{R_i/R}=\sqrt{RR_i}$ (where we assume $ R=R_{i-1}$). Finally, the velocity waves in section $ i-1$ can be scaled to equal their corresponding force waves by introducing a transformer $ g=\sqrt{1/R}$ on the left, which then coincides Eq.$ \,$(C.126) (but with a minus sign in the second equation).

Next Section:
The Digital Waveguide Oscillator
Previous Section:
FDNs as Digital Waveguide Networks