DSPRelated.com
Free Books
   Free Books    Physical Audio Signal Processing  

Mass Termination Model

The previous discussion solved for the motion of an ideal mass striking an ideal string of infinite length. We now investigate the same model from the string's point of view. As before, we will be interested in a digital waveguide (sampled traveling-wave) model of the string, for efficiency's sake (Chapter 6), and we therefore will need to know what the mass ``looks like'' at the end of each string segment. For this we will find that the impedance description (§7.1) is especially convenient.

Figure 9.15: Physical model of mass-string collision after time 0. The mass is drawn as having a finite diameter for conceptual clarity. However, the model is formulated for the limit as the diameter approaches zero in the figure (bringing all three forces together to act on a single mass-string junction point). In other words, we assume a point mass.
\includegraphics[width=\twidth]{eps/massstringphynum}

Let's number the string segments to the left and right of the mass by 1 and 2, respectively, as shown in Fig.9.15. Then Eq.$ \,$(9.8) above may be written

$\displaystyle 0 \eqsp f_m(t) + f_{1m}(t) + f_{2m}(t), \protect$ (10.11)

where $ f_{1m}(t)$ denotes the force applied by string-segment 1 to the mass (defined as positive in the ``up'', or positive-$ y$ direction), $ f_{2m}(t)$ is the force applied by string-segment 2 to the mass (again positive upwards), and $ f_m(t)$ denotes the inertial force applied by the mass to both string endpoints (where again, a positive force points up).

To derive the traveling-wave relations in a digital waveguide model, we want to use the force-wave variables $ f_1=f^{{+}}_1+f^{{-}}_1$ and $ f_2=f^{{+}}_2+f^{{-}}_2$ that we defined for vibrating strings in §6.1.5; i.e., we defined $ f_i\isdeftext -Ky'_i$, where $ K$ is the string tension and $ y'_i$ is the string slope, $ i=1,2$.

Figure 9.16: Depiction of a string segment with negative slope (center), pulling up to the right and down to the left. (Horizontal force components are neglected.)
\includegraphics[width=0.5\twidth]{eps/stringslope}

As shown in Fig.9.16, a negative string slope pulls ``up'' to the right. Therefore, at the mass point we have $ f_{1m}(t) = f_1(t,x_m) = -Ky'_1(t,x_m)$, where $ x_m$ denotes the position of the mass along the string. On the other hand, the figure also shows that a negative string slope pulls ``down'' to the left, so that $ f_{2m}(t) = -f_2(t,x_m) = Ky'_2(t,x_m)$. In summary, relating the forces we have defined for the mass-string junction to the force-wave variables in the string, we have

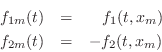
\begin{eqnarray*} f_{1m}(t) &=& \quad\! f_1(t,x_m)\\ f_{2m}(t) &=& -f_2(t,x_m) \end{eqnarray*}

where $ x_m$ denotes the position of the mass along the string.

Thus, we can rewrite Eq.$ \,$(9.11) in terms of string wave variables as

$\displaystyle 0 \eqsp f_m(t) + f_{1}(t,x_m) - f_{2}(t,x_m), \protect$ (10.12)

or, substituting the definitions of these forces,

$\displaystyle 0 \eqsp -m\dot v(t) - K\,y'_1(t,0) + K\, y'_2(t,0). \protect$ (10.13)

The inertial force of the mass is $ f_m(t)=-m\dot v(t)$ because the mass must be accelerated downward in order to produce an upward reaction force. The signs of the two string forces follow from the definition of force-wave variables on the string, as discussed above.

The force relations can be checked individually. For string 1,

$\displaystyle m\dot v(t) + K\,y'_1(t,0) = 0 $

states that a positive slope in the string-segment to the left of the mass corresponds to a negative acceleration of the mass by the endpoint of that string segment. Similarly, for string 2,

$\displaystyle m\dot v(t) - K\,y'_2(t,0) = 0 $

says that a positive slope on the right accelerates the mass upwards. Similarly, a negative slope pulls ``up'' to the right and ``down'' to the left, as shown in Fig.9.16 above.

Now that we have expressed the string forces in terms of string force-wave variables, we can derive digital waveguide models by performing the traveling-wave decompositions $ f_1=f^{{+}}_1+f^{{-}}_1$ and $ f_2=f^{{+}}_2+f^{{-}}_2$ and using the Ohm's law relations $ f^{{+}}_i=Rv^{+}_i$ and $ f^{{-}}_i=-Rv^{-}_i$ for $ i=1,2$ (introduced above near Eq.$ \,$(6.6)).

Next Section:
Mass Reflectance from Either String
Previous Section:
Matrix Bridge Impedance