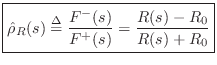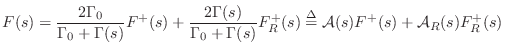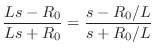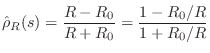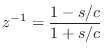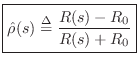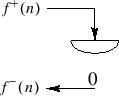Wave Digital Elements
When modeling mechanical systems composed of masses, springs, and dashpots, it is best to begin with an electrical equivalent circuit. Equivalent circuits make clear the network-theoretic structure of the system, clearly indicating, for example, whether interacting elements should be connected in series or parallel. Each element of the equivalent circuit can then be replaced by a first-order wave digital element, and the elements are finally parallel or series connected by means of scattering-junction interfaces known as adaptors.
Wave digital elements may be derived from their describing differential equations (in continuous time) as follows:
- First express
all physical quantities (such as force and velocity) in terms of
traveling-wave components. The traveling wave components are called
wave variables. For example, the force
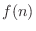 on a mass is
decomposed as
on a mass is
decomposed as
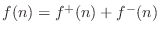 , where
, where
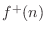 is regarded as a
traveling wave propagating toward the mass, while
is regarded as a
traveling wave propagating toward the mass, while
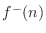 is
seen as the traveling component propagating
away from the mass. A ``traveling wave'' view of
force mediation is actually much closer to physical reality than any
instantaneous model.
is
seen as the traveling component propagating
away from the mass. A ``traveling wave'' view of
force mediation is actually much closer to physical reality than any
instantaneous model.
- Second, digitize the resulting traveling-wave system using the
bilinear transform. The bilinear transform is equivalent in
the time domain to the
trapezoidal rule for numerical integration (see §7.3.2).
- Connect
 elementary units together by means of
elementary units together by means of
 -port scattering junctions. There are two basic
types of scattering junction, one for parallel, and one for series
connection. (See §C.8 for the theory of scattering junctions.)
-port scattering junctions. There are two basic
types of scattering junction, one for parallel, and one for series
connection. (See §C.8 for the theory of scattering junctions.)
An important benefit of introducing wave variables prior to bilinear transformation is the elimination of delay-free loops when connecting elementary building blocks. In other words, any number of elementary models can be interconnected, in series or in parallel, and the resulting finite-difference scheme remains explicit (free of delay-free loops).
A Physical Derivation of Wave Digital Elements
This section provides a ``physical'' derivation of Wave Digital Filters (WDF), which contrasts somewhat with the more formal derivation common in the literature. The derivation is presented as a numbered series of steps (some with rather long discussions):
- To each element, such as a capacitor or inductor, attach a
length of waveguide (electrical transmission line) having wave
impedance
 , and make it infinitesimally long. (Take the limit as
its length goes to zero.) A schematic depiction of this is shown in
Fig.F.1a. For consistency, all signals are Laplace transforms of
their respective time-domain signals. The length must approach zero
in order not to introduce propagation delays into the signal path.
, and make it infinitesimally long. (Take the limit as
its length goes to zero.) A schematic depiction of this is shown in
Fig.F.1a. For consistency, all signals are Laplace transforms of
their respective time-domain signals. The length must approach zero
in order not to introduce propagation delays into the signal path.
Figure F.1: a) Physical schematic for the derivation of a wave digital model of driving-point impedance 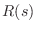 . The inserted
waveguide impedance
. The inserted
waveguide impedance  is real and positive, but otherwise
arbitrary. b) Expanded view of the interior of the infinitesimal
waveguide section, also representing the termination impedance
is real and positive, but otherwise
arbitrary. b) Expanded view of the interior of the infinitesimal
waveguide section, also representing the termination impedance 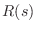 as an impedance-step within the waveguide.
as an impedance-step within the waveguide.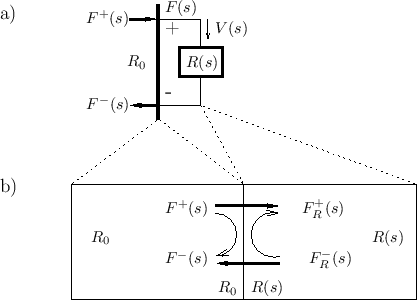
Points to note:
- The infinitesimal waveguide is terminated by the element.
The element reflects waves as if it were a new waveguide section at
impedance
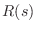 , as depicted in Fig.F.1b.
, as depicted in Fig.F.1b.
- The interface to the element is recast as traveling-wave
components
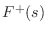 and
and 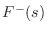 at impedance
at impedance  .
In terms of these components, the physical force on the element is
obtained by adding them together:
.
In terms of these components, the physical force on the element is
obtained by adding them together:
 .
.
- The waveguide impedance
 is arbitrary because it
has been physically introduced. We will need to know it when we
connect this element to other elements. The element's interface to
other elements is now a waveguide (transmission line) at real
impedance
is arbitrary because it
has been physically introduced. We will need to know it when we
connect this element to other elements. The element's interface to
other elements is now a waveguide (transmission line) at real
impedance  .
.
- The junction is ``parallel'' (cf. §7.2):
- Force (voltage) must be continuous across the junction, since
otherwise there would be a finite force across a zero mass, producing
infinite acceleration.
- The sum of velocities (currents) into the junction must be zero
by conservation of mass (charge).
- Force (voltage) must be continuous across the junction, since
otherwise there would be a finite force across a zero mass, producing
infinite acceleration.
- The infinitesimal waveguide is terminated by the element.
The element reflects waves as if it were a new waveguide section at
impedance
Reflectance of a General Lumped Waveguide Termination
Calculate the reflectance of the terminated waveguide. That is, find the Laplace transform of the return wave divided by the Laplace transform of the input wave going into the waveguide. In general, the reflectance of an impedance step for force waves (voltage waves in the electrical case) is
This is easily derived from continuity constraints across the junction. Specifically, referring to Fig.F.1b, let

By the definition of wave impedance in a waveguide, we have
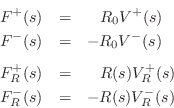
Thus,
![\begin{eqnarray*}
0 &=& V(s) + V_R(s)\\
&=& \left[V^{+}(s)+V^{-}(s)\right] + ...
...s)}\right]
&=& \frac{2}{R_0}F^{+}(s) + \frac{2}{R(s)}F^{+}_R(s)
\end{eqnarray*}](http://www.dsprelated.com/josimages_new/pasp/img4758.png)
Defining
![]() and
and
![]() , we have
, we have
Now that we've solved for the junction force
Finally, the force-wave reflectance of an impedance step from
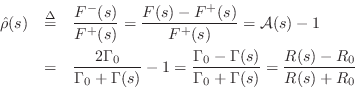
as claimed.
Reflectances of Elementary Impedances
We now derive the reflectances of the elements used in LTI analog electric circuits, viz., the capacitor, inductor, and resistor.
Capacitor Reflectance
For a capacitor of ![]() Farads, the driving-point impedance is (see
§7.1.3)
Farads, the driving-point impedance is (see
§7.1.3)
Inductor Reflectance
For an inductor of ![]() Henrys, we have
Henrys, we have
Resistor Reflectance
Finally, for a resistor of ![]() Ohms, we get
Ohms, we get
Note that both the capacitor and inductor reflectances are
stable allpass filters, as they must be. Also, the resistor
reflectance is always less than 1, no matter what waveguide impedance
![]() we choose.
we choose.
Choosing Impedance to Simplify Element Reflectance
Observe that there is a natural choice for each waveguide impedance which will give us a normalized, ``universal reflectance'' for each element:
- For the capacitor, setting
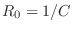 gives
gives
- For the inductor, setting
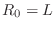 gives
gives
- And for the resistor, we set
 to obtain
to obtain
Digitizing Elementary Reflectances by Bilinear Transform
Going to discrete time via the bilinear transform means making the substitution
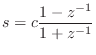 |
(F.11) |
where
Solving for ![]() gives us the inverse bilinear transform:
gives us the inverse bilinear transform:
In this case, we see that setting ![]() further simplifies our
universal reflectances in the digital domain:
further simplifies our
universal reflectances in the digital domain:
- For the ``wave digital capacitor'' (or spring), Eq.
 (F.8) becomes
(F.8) becomes

- For the ``wave digital inductor'' (or mass), Eq.
 (F.9) becomes
(F.9) becomes
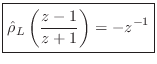
- And for the ``wave digital resistor'' (or dashpot), Eq.
 (F.10) becomes
as before in the continuous-time case.
(F.10) becomes
as before in the continuous-time case.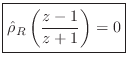
Note that this choice of ![]() is also the only one that eliminates
delay-free paths in the fundamental elements. This allows them to
be used as building blocks for explicit finite difference
schemes.
is also the only one that eliminates
delay-free paths in the fundamental elements. This allows them to
be used as building blocks for explicit finite difference
schemes.
We may still obtain the above results using the more typical value
![]() (instead of
(instead of ![]() ) in the bilinear transform. From
Eq.
) in the bilinear transform. From
Eq.![]() (F.12), it is clear that changing
(F.12), it is clear that changing ![]() amounts to a linear
frequency scaling of
amounts to a linear
frequency scaling of ![]() . Such a scaling may be compensated
by choosing the waveguide (port) impedances to be
. Such a scaling may be compensated
by choosing the waveguide (port) impedances to be
![]() (instead of
(instead of ![]() ) for the inductor, and
) for the inductor, and
![]() (instead of
(instead of
![]() ) for the capacitor.
) for the capacitor.
Summary of Wave Digital Elements
From Eq.![]() (F.1), we have that the general reflectance of impedance
(F.1), we have that the general reflectance of impedance
![]() with respect to the reference impedance
with respect to the reference impedance ![]() in the wave
variable formulation is given by
in the wave
variable formulation is given by
In WDF construction, the free constant in the bilinear transform is taken to be
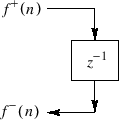
Wave Digital Mass
In the case of a mass ![]() , we have
, we have
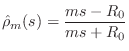
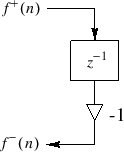 |
Thus, the wave digital mass is simply a unit-sample delay and a negation. The fact that the value of the mass has been canceled out will be addressed below in the subsection on ``adaptors,'' i.e., it only affects interconnection with other elements. For now, just remember that the reference impedance was chosen to be equal to the mass in order to get this simple wave flow diagram. Also note that the WDF mass simulator has no delay-free path from input to output.
Wave Digital Spring
In the case of a spring with stiffness ![]() , we have the impedance
, we have the impedance
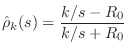
![$\displaystyle \fbox{$\displaystyle \hat{\tilde{\rho}}_k(z) = z^{-1}$} \qquad\makebox[0pt][l]{(Wave Digital Spring)}
$](http://www.dsprelated.com/josimages_new/pasp/img4811.png)
Thus, the WDF of a spring is simply a unit-sample delay, which is just the negative of the WDF mass. If we were to switch to velocity waves instead of force waves, both masses and springs would again correspond to unit-sample delays, but the spring would become inverting and the mass non-inverting.
Wave Digital Dashpot
Starting with a dashpot with coefficient ![]() , we have
, we have
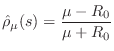
In the context of waveguide theory, a zero reflectance corresponds to a matched impedance, i.e., the terminating transmission-line impedance equals the characteristic impedance of the line.
The difference equation for the wave digital dashpot is simply
![]() . While this may appear overly degenerate at first,
remember that the interface to the element is a port at impedance
. While this may appear overly degenerate at first,
remember that the interface to the element is a port at impedance
![]() . Thus, in this particular case only, the infinitesimal
waveguide interface is the element itself.
. Thus, in this particular case only, the infinitesimal
waveguide interface is the element itself.
Limiting Cases
The force-wave reflectance of an infinite impedance (rigid wall or ``open circuit'') is
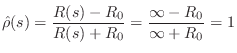
Similarly, the force-wave reflectance of a zero impedance (free termination, frictionless surface, or ``short circuit'') is
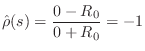
For velocity waves, we obtain the opposite results: rigid terminations are inverting, and free terminations are non-inverting.
Unit Elements
The unit element two-port is simply a bidirectional delay line with half a sample delay in each direction. As a result, it really belongs under the topic of distributed modeling. To avoid delay-free loops, Fettweis noted [135] that every pair of adaptors must be separated by at least one unit element. More recently, this objective is accomplished instead using ``reflection-free ports'' [136] (see also §F.2.2).
Next Section:
Adaptors for Wave Digital Elements
Previous Section:
Acknowledgments