Launch of Youtube Channel: My First Videos - Embedded World 2017
I went to Embedded World 2017 in Nuremberg with an ambitious plan; I would make video highlights of several exhibits (booths) to be presented to the *Related sites audience. I would try to make the vendors focus their pitch on the essential...
A Two Bin Exact Frequency Formula for a Pure Complex Tone in a DFT
●1 commentIntroduction This is an article to hopefully give a better understanding to the Discrete Fourier Transform (DFT) by deriving an exact formula for the frequency of a complex tone in a DFT. It is basically a parallel treatment to the real case...
DFT Bin Value Formulas for Pure Complex Tones
Introduction This is an article to hopefully give a better understanding to the Discrete Fourier Transform (DFT) by deriving an analytical formula for the DFT of pure complex tones and an alternative variation. It is basically a parallel...
Multi-Decimation Stage Filtering: Design and Optimization
●1 commentDuring my research on digital FIR decimation filters I have been developing various Matlab scripts and functions. In which I have decided later on to consolidate it in a form of a toolbox. I have developed this toolbox to assist and...
Canonic Signed Digit (CSD) Representation of Integers
●1 commentIn my last post I presented Matlab code to synthesize multiplierless FIR filters using Canonic Signed Digit (CSD) coefficients. I included a function dec2csd1.m (repeated here in Appendix A) to convert decimal integers to binary CSD...
Frequency Translation by Way of Lowpass FIR Filtering
●1 commentSome weeks ago a question appeared on the dsp.related Forum regarding the notion of translating a signal down in frequency and lowpass filtering in a single operation [1]. It is possible to implement such a process by embedding a discrete cosine...
Minimum Shift Keying (MSK) - A Tutorial
●7 commentsMinimum Shift Keying (MSK) is one of the most spectrally efficient modulation schemes available. Due to its constant envelope, it is resilient to non-linear distortion and was therefore chosen as the modulation technique for the GSM cell phone...
Round Round Get Around: Why Fixed-Point Right-Shifts Are Just Fine
●1 commentToday’s topic is rounding in embedded systems, or more specifically, why you don’t need to worry about it in many cases.One of the issues faced in computer arithmetic is that exact arithmetic requires an ever-increasing bit length to...
Some Thoughts on Sampling
●1 commentSome time ago, I came across an interesting problem. In the explanation of sampling process, a representation of impulse sampling shown in Figure 1 below is illustrated in almost every textbook on DSP and communications. The question is: how is...
Matlab Code to Synthesize Multiplierless FIR Filters
This article presents Matlab code to synthesize multiplierless Finite Impulse Response (FIR) lowpass filters. A filter coefficient can be represented as a sum of powers of 2. For example, if a coefficient = decimal 5 multiplies input x,...
A Fixed-Point Introduction by Example
●8 commentsIntroduction The finite-word representation of fractional numbers is known as fixed-point. Fixed-point is an interpretation of a 2's compliment number usually signed but not limited to sign representation. It...
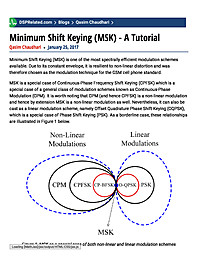
Minimum Shift Keying (MSK) - A Tutorial
●7 commentsMinimum Shift Keying (MSK) is one of the most spectrally efficient modulation schemes available. Due to its constant envelope, it is resilient to non-linear distortion and was therefore chosen as the modulation technique for the GSM cell phone...
Polyphase Filters and Filterbanks
●2 commentsALONG CAME POLY Polyphase filtering is a computationally efficient structure for applying resampling and filtering to a signal. Most digital filters can be applied in a polyphase format, and it is also possible to create efficient resampling...
Polyphase filter / Farrows interpolation
●5 commentsHello, this article is meant to give a quick overview over polyphase filtering and Farrows interpolation. A good reference with more depth is for example Fred Harris' paper: http://www.signumconcepts.com/IP_center/paper018.pdf The task is as...
Understanding and Relating Eb/No, SNR, and other Power Efficiency Metrics
●7 commentsIntroduction Evaluating the performance of communication systems, and wireless systems in particular, usually involves quantifying some performance metric as a function of Signal-to-Noise-Ratio (SNR) or some similar measurement. Many systems...
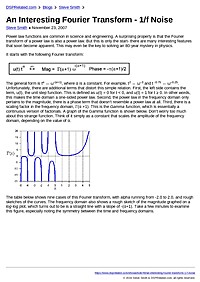
An Interesting Fourier Transform - 1/f Noise
●4 commentsPower law functions are common in science and engineering. A surprising property is that the Fourier transform of a power law is also a power law. But this is only the start- there are many interesting features that soon become apparent. This may...
Handling Spectral Inversion in Baseband Processing
●3 commentsThe problem of "spectral inversion" comes up fairly frequently in the context of signal processing for communication systems. In short, "spectral inversion" is the reversal of the orientation of the signal bandwidth with...
Understanding and Implementing the Sliding DFT
●4 commentsIntroduction In many applications the detection or processing of signals in the frequency domain offers an advantage over performing the same task in the time-domain. Sometimes the advantage is just a simpler or more conceptually...
Wavelets II - Vanishing Moments and Spectral Factorization
●1 commentIn the previous blog post I described the workings of the Fast Wavelet Transform (FWT) and how wavelets and filters are related. As promised, in this article we will see how to construct useful filters. Concretely, we will find a way to calculate...
Linear-phase DC Removal Filter
●1 commentThis blog describes several DC removal networks that might be of interest to the dsprelated.com readers. Back in August 2007 there was a thread on the comp.dsp newsgroup concerning the process of removing the DC (zero Hz) component from a...
